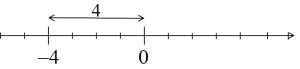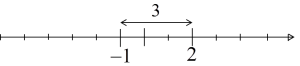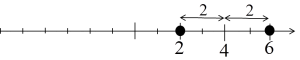A Few Things You Should Learn Well in High School But No One Told You – Part 1 Absolute Values

---

If you’ve been studying mathematics at university for a while now, you’ve probably noticed that most of the material covered in high school doesn’t directly help you at university. If you’re just starting out – take note of this.

What does “directly” mean?

I say “directly” because obviously every drop of your mathematical sweat in high school won’t be wasted and will be counted for you. I’m talking about practicing abstract thinking, the ability to concentrate on a problem, and so on.

However, frankly speaking, you won’t need to go back to many of the high school math topics, even to just take a peek and revise them.

But there are exceptions.

There are things covered in high school, without which you won’t get far in university.

At the beginning of the academic year, it’s worth revisiting or simply learning them. This way you’ll pave the way for further, new topics. If not covered, they will constantly nag at you, like a thorn in your shoe, making it difficult (or even impossible) to move forward.

These won’t be entire large sections of mathematics, but rather small details, little bricks, without which the whole structure might collapse.

Definition of a Limit

Take, for example, the definition of the limit of a sequence, which you’ll encounter very soon:

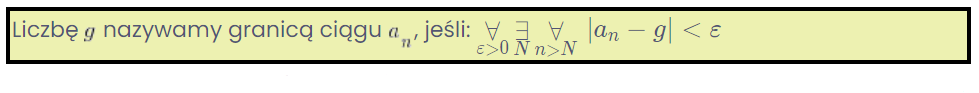
Do you understand these weird symbols on the left? And this absolute value on the right? Not much?

And yet, to understand or even correctly read this definition, knowing quantifiers (those creatures on the left: ) and the geometric interpretation of absolute value (on the right) from high school should be enough…

But let’s not complain, let’s get to work. In this and the next posts, I’ll show you a few details, understanding which will greatly ease your university studies.

Let’s start with…

1. Geometric Interpretation of Absolute Value (as Distance)

We all know roughly what this absolute value is about. Nobody has a problem with the fact that:

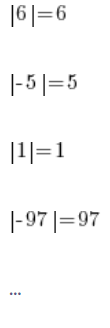
The operation of absolute value simply returns the same number, and if it is negative, changing its sign to plus.

What would it mean geometrically? On the number line? Well, simply the distance of a number from zero. For example, 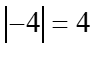and indeed, without a doubt, the distance of the number from zero is 4:

We will continue to follow this trail and understand absolute value precisely as distance.

Let’s notice one more thing:

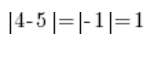
And that on the number line:

…that is, the distance between 4 and 5 equals the numerical value of the DIFFERENCE (subtraction) of 4 and 5, regardless of what we subtract from what, because:

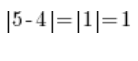
Similarly, if we subtract numbers 3 and 8 and calculate the absolute value of the result, we will get exactly the distance on the number line between numbers 3 and 8 (it will be 5).

This also works with negative numbers, for example -1 and 2:

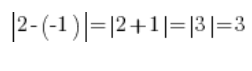
…that is, precisely the distance between numbers -1 and 2:

You can also play around with this a bit yourself. If you have a high school textbook, I strongly encourage you to sit down for an hour on this topic and mess around a bit.

We can formulate a general rule:

The absolute value of the difference (subtraction) of two numbers: and equals the distance between these numbers.

So how can we decipher, for example, such an equation:

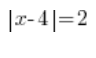
We know that the part means the distance of the unknown x from 4, and on the right side of the equality we know that this distance should be equal to 2.

So the solution to the equation will be numbers whose distance from 4 is 2, i.e., numbers 2 and 6:

And now let’s take this inequality:

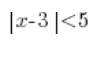
Its left side is the distance x from number 3, and reading further we get the information that this distance should be less than 5. This time the solution to this inequality will not be just two numbers but many more – all numbers whose distance from 3 is less than 5. On the axis, it would look like this:

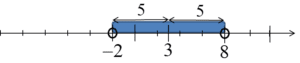
…that is, it would be all numbers from -2 to 8, that is, the numerical interval: . The distance of each such number from 3 is less than 5.

This numerical interval can also be called a certain neighborhood of point 3 (an important term, often used in university).

By reasoning in the same way, you can figure out which numbers satisfy the inequality …

However, this is not really my goal (you had this in high school, right 🙂 ?).

It is good to understand absolute value as distance, because it appears in many mathematical definitions you will encounter in university. And then it’s good to know right away, “what’s going on”.

For example, let’s take the previously mentioned limit of a sequence:

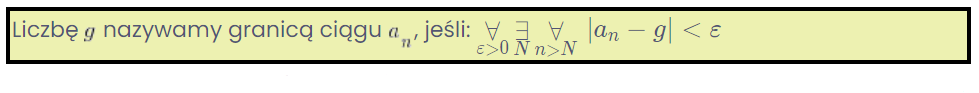
Let’s focus for now on the right side of the formula. Let’s leave the weird symbols on the left for the next post.

Which reading hits more to the heart and is better understood:

“the absolute value of the difference between and must be less than ”

or:

“the distance between and must be less than ”

?

Formally, both ways of phrasing the definition are equivalent, but the second is simpler and focuses on the essence, without being distracted by some arithmetic operations like difference.

Understanding absolute value as distance will make it much easier for you to understand many seemingly complex mathematical theorems and definitions in university.

What’s next?

In the next post, I will deal with these weird symbols: and , so we will be able to fully understand our example definition.