 Professors at universities have their own requirements. Many of them – for the good of their students, of course – won’t hesitate to specify in great detail the rules on how tasks should be solved.
Professors at universities have their own requirements. Many of them – for the good of their students, of course – won’t hesitate to specify in great detail the rules on how tasks should be solved.
A user of my Indefinite Integrals Course wrote to me on GG like this:
I have a request, could you show on your FB or blog how integrals in your formulas are derived to the form on the paper? I mean formulas No.: 5, 9, 10, 13, 14, 15, 16. Unfortunately, our professor told us that only the simplest ones can be used, the more complex ones I mentioned need to be broken down to the given form by ourselves. I think many people would be grateful to you 🙂
He means the formula sheet attached to the Course:
Formulas for Indefinite Integrals
And specifically these formulas:
5.\quad \int{{{a}^{x}}dx=\frac{{{a}^{x}}}{\ln a}+C}9.\quad \int{tgxdx=-\ln \left| \cos x \right|+C}
10.\quad \int{ctgxdx=\ln \left| \sin x \right|}+C
13.\quad \int{\frac{dx}{{{x}^{2}}+{{a}^{2}}}=\frac{1}{a}arctg\frac{x}{a}+C}
14.\quad \int{\frac{dx}{{{x}^{2}}-{{a}^{2}}}=\frac{1}{2a}\ln \left| \frac{x-a}{x+a} \right|+C}
15.\quad \int{\frac{dx}{\sqrt{{{a}^{2}}-{{x}^{2}}}}=\arcsin \frac{x}{a}+C}
16.\quad \int{\frac{dx}{\sqrt{{{x}^{2}}+q}}=\ln \left| x+\sqrt{{{x}^{2}}+q} \right|+C}
If not these, then others
Yes, it’s true, professors often require using certain formulas and not others. Or not using some formulas at all. Or using those we don’t like to use.
The only reasonable way in such situations is complete compliance. In the exam hall, the professor is the law, and there’s no point in complaining to friends later that the professor didn’t pass the exam, even though “he should have”.
Instead, I will look at the mentioned formulas one by one and show how to deal with each case individually (unfortunately, they can’t be “embraced” by any common rule). “Deal with” means solving integrals requiring the use of that formula without using that formula – but using a less general formula, or deriving the integral through substitution, or rational method.
So, one by one:
5.\quad \int{{{a}^{x}}dx=\frac{{{a}^{x}}}{\ln a}+C}
With this formula, I don’t really know what the issue is, it directly follows from reversing the derivative formula:
{{\left( {{a}^{x}} \right)}^{\prime }}={{a}^{x}}\ln aSo here I don’t show complete submission to the professor, but ask for an explanation of how to calculate \int{{{3}^{x}}dx} without using the formula \int{{{a}^{x}}dx}.
If anyone comes up with an interesting idea, please share it with humanity in the comments below the post.
9.\quad \int{tgxdx=-\ln \left| \cos x \right|+C}
Alright, back to the game.
This formula doesn’t directly follow from reversing any derivative formula.
If we agree that we don’t know it, we can calculate the integral \int{tgxdx} by substitution:
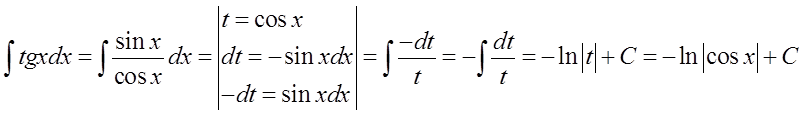
Here, similarly to the previous one:
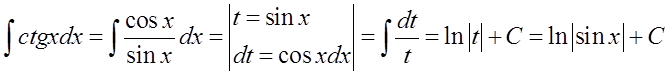
This formula is a general form of the formula:
\int{\frac{dx}{{{x}^{2}}+1}=arctgx+C} or: \int{\frac{dx}{1+{{x}^{2}}}=arctgx+C}
The professor wants us to use the formula: \int{\frac{dx}{{{x}^{2}}+1}=arctgx+C} (resulting from a simple reversal of the derivative formula), and not use the formula: \int{\frac{dx}{{{x}^{2}}+{{a}^{2}}}=\frac{1}{a}arctg\frac{x}{a}+C} (which is already a “processed” formula).
We do this as follows (by transformation and substitution):
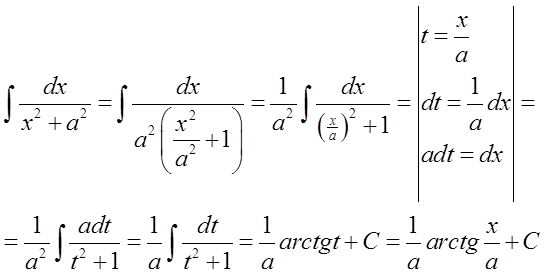
In a specific example, it might look like this:

This formula is different from the previous one, it doesn’t require using a formula where ‘a’ is replaced by ‘1’ (such a formula doesn’t exist). An alternative to using this formula is to decompose into partial fractions as in rational integrals (I showed how to do this in Lesson 5 of the Indefinite Integrals Course).
In fact, \frac{1}{{{x}^{2}}-{{a}^{2}}}=\frac{1}{\left( x-a \right)\left( x+a \right)} and can be further decomposed into partial fractions. For example:
\frac{1}{{{x}^{2}}-9}=\frac{1}{\left( x-3 \right)\left( x+3 \right)} \frac{1}{\left( x-3 \right)\left( x+3 \right)}=\frac{A}{x-3}+\frac{B}{x+3}Then we multiply by \left( x-3 \right)\left( x+3 \right), calculate constants A, B by comparing polynomials and everything as shown in Lesson 5 of the Course.
15.\quad \int{\frac{dx}{\sqrt{{{a}^{2}}-{{x}^{2}}}}=\arcsin \frac{x}{a}+C}Here again, the general formula: \int{\frac{dx}{\sqrt{{{a}^{2}}-{{x}^{2}}}}=\arcsin \frac{x}{a}+C} should be transformed into the specific formula: \int{\frac{dx}{\sqrt{1-{{x}^{2}}}}=\arcsin x+C}.
We do this similarly to formula 13):
In a specific example, it might look like this:
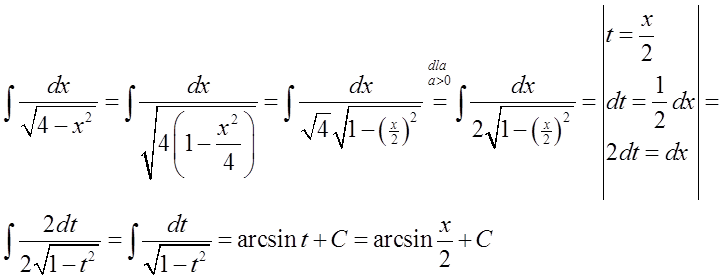
The matter is more complicated, requiring the use of so-called “hyperbolic substitutions” (hyperbolic sine and cosine). I will leave this topic in this post, and will certainly write about these substitutions soon.
These are the formulas the user asked about, and I will add that the formulas added to the list of basic formulas by me:
\int{{{e}^{ax}}dx}=\frac{1}{a}{{e}^{ax}}+C \int{\sin axdx}=-\frac{1}{a}\cos ax+C \int{\cos axdx}=\frac{1}{a}\sin ax+CAre derived through simple substitution: t=ax
So, for example, having the integral: \int{{{e}^{-x}}dx} and NOT being able to use (due to the professor’s preferences) the formula \int{{{e}^{ax}}dx}=\frac{1}{a}{{e}^{ax}}+C, we use the substitution t=-x and calmly continue the calculation.

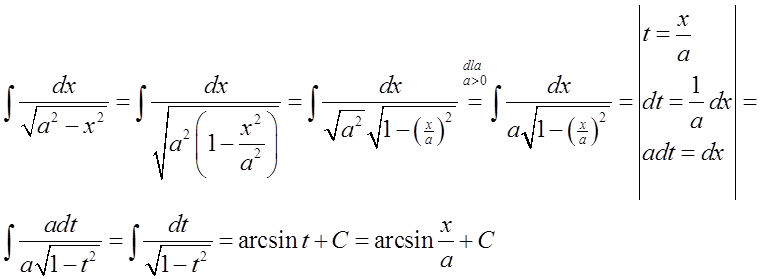

2 Comments
Sistem Informasi Akuntansi
What request did the user of the Indefinite Integrals Course make regarding the formulas provided in the course material? Greeting : IT Telkom
Krystian Karczyński
He requested help in solving indefinite integrals without using formulas number 5, 9, 10, 13, 14, 15, and 16 from the formula sheet included in my course:
Formulas for Indefinite Integrals
The professor in class prohibited students from using these formulas.